This chapter covers:
- What cryptography is about.
- The difference between theoretical cryptography and real-world cryptography.
- What you will learn throughout this adventure.
Greetings traveler,
Sit tight as you’re about to enter a world of wonder and mystery—the world of cryptography. Cryptography is the ancient discipline of securing situations that are troubled with malicious characters. In this book are the spells we need to defend ourselves against the malice. Many have attempted to learn this craft, but few have survived the challenges that stand in the way of mastery. Exciting adventures await! We’ll uncover how cryptographic algorithms can secure our letters, identify our allies, and protect treasures from our enemies. Sailing through the cryptographic sea will not be the smoothest journey, as cryptography is the foundation of all security in our world, the slightest mistake could be deadly.
Remember
If you find yourself lost, keep moving forward. It will all eventually make sense.
highlight, annotate, and bookmark
You can automatically highlight by performing the text selection while keeping the alt/ key pressed.

Our journey starts here with an introduction to cryptography, the science aiming to defend protocols against saboteurs. But first, what’s a protocol? Simply put: it’s a list of steps that one (or more people) must follow in order to achieve something. For example, imagine the following premise: you want to leave your magic sword unattended for a few hours so you can take a nap. One protocol to do this could be the following:
- Deposit weapon on the ground.
- Take nap under a tree.
- Recover weapon from the ground.
Of course, it’s not a great protocol as anybody can steal your sword while you’re napping… And so cryptography is about taking into account these adversaries who are looking to take advantage of you.
In ancient times, when rulers and generals were busy betraying each other and planning coups, one of their biggest problems was finding a way to share confidential information with those they trusted. From here the idea of cryptography was born. It took centuries and hard work before cryptography became the serious discipline it is today. Now, it’s used all around us, to provide the most basic services in the face of our chaotic and adverse world.
The story of this book is about the practice of cryptography on our planet. It takes you on an expedition throughout the computing world to cover cryptographic protocols in use today, it shows you what are the parts they are made of, and how everything fits together. While your typical cryptography book usually starts with the discovery of cryptography, and takes you through its history, I think that it makes little sense for me to kick off things this way. I want to tell you about the practical. I want to tell you about what I’ve witnessed myself, reviewing cryptographic applications for large companies as a consultant, or the cryptography I’ve made use of myself as an engineer in the field.
There will be (almost) no scary math formulas. The purpose of this book is to demystify cryptography, survey what is considered useful nowadays, and give intuition about how things around you are built. This book is intended for the curious people, the interested engineers, the adventurous developers and the inquisitive researchers.
Chapter 1 initiates a tour of the world of cryptography. We will discover the different types of cryptography, which ones matter to us, and how the world agreed on using them.
discuss
One of the fundamental concepts of cryptography is symmetric encryption. It is used in a majority of cryptographic algorithms in this book, and it is thus extremely important. We introduce this new concept here via our very first protocol. Let’s imagine that queen Alice needs to send a letter to lord Bob who is a few castles away. She asks her loyal messenger to ride his camel and battle his way through the filthy lands ahead in order to deliver the precious message to lord Bob. Yet, she is suspicious; even though her loyal messenger has served her for many years, she wishes the message in transit to remain secret from all passive observers, including the messenger. You see, the letter most likely contains some controversial gossip about the kingdoms on the way.

What queen Alice needs is a protocol that acts like she would effectively hand the message to lord Bob herself. No middle men. This is quite an impossible problem to solve in practice, unless we introduce cryptography (or teleportation) into the equation. And this is what we ended up doing ages ago by inventing a new type of cryptographic algorithm called a symmetric encryption algorithm (also known as a cipher).
![[Note]](https://drek4537l1klr.cloudfront.net/wong/v-12/Figures/note.png) Note
Note
By the way, a type of cryptographic algorithm is often referred to as a primitive. You can think of a primitive as the smallest useful construction you can have in cryptography, and it is often used with other primitives in order to build a protocol. It is mostly a term, and has no particularly important meaning, though it appears often enough in the literature that it is good to know about it.
Let’s see next how an encryption primitive can be used to hide queen Alice’s message from the messenger. Imagine for now that the primitive is a black box (we can’t see what it’s doing internally) that provides two functions:
-
ENCRYPT
-
DECRYPT
The first function, ENCRYPT works by taking a secret key (usually a very large number) and a message. It then outputs a series of numbers that look like they were chosen randomly, some noisy data if you will. We will call that output the encrypted message. I illustrate this in figure 1.1.
Figure 1.1. The ENCRYPT function takes a message and a secret key, and outputs the encrypted message—a long series of numbers that look like random noise.

The second function, DECRYPT, is the inverse of the first one: by taking the same secret key and the random output of the first function (the encrypted message), it finds back the original message.
Figure 1.2. The DECRYPT function takes an encrypted message and a secret key, and returns the original message.

To make use of this new primitive, queen Alice and lord Bob have to first meet in real life and decide on what secret key to use. Later, queen Alice can use the provided ENCRYPT function to protect a message with the help of the secret key. She then passes the encrypted message to her messenger, who eventually delivers it to lord Bob. Lord Bob then uses the DECRYPT function on the encrypted message, with the same secret key, to recover the original message.
Figure 1.3. (1) Alice uses the ENCRYPT function with a secret key to transform her message into noise. (2) She then passes the encrypted message to her messenger, who will not learn anything about the underlying message. (3) Once Bob receives the encrypted message, he can recover the original content by using the DECRYPT function with the same secret key Alice used.

During this exchange, all the messenger had was something that looked random and that provided no meaningful insight into the content of the hidden message. Effectively, we augmented our insecure protocol into a secure one thanks to the help of cryptography. The new protocol makes it possible for queen Alice to deliver a confidential letter to lord Bob without anyone (but lord Bob) learning the content of it. The process of using a secret key to render things to noise, making them indistinguishable from random, is a common way of securing a protocol in cryptography. We will see more of this as we learn more cryptographic algorithms in the next chapters.
By the way, symmetric encryption is part of a larger category of cryptography algorithms called symmetric cryptography or secret-key cryptography. This is due to the same key being used by the different functions exposed by the cryptographic primitive. As you will see later, sometimes there are more than one key.
settings

To design a cryptographic algorithm (like our encryption primitive) is an easy task, but to design a secure cryptographic algorithm is not for the faint of heart. While we shy away from creating such algorithms in this book, we do learn how to recognize the good ones to use. This can be difficult, as there is more choice than one can ask for the task. Hints can be found in the repeated failures of the history of cryptography, as well as the lessons that the community has learned from them. As we take a look at the past, we will grasp at what turns a cryptographic algorithm into a trusted-to-be-secure one.
Hundreds of years have passed and many queens and lords have been buried. Since then, paper has been abandoned as our primary means of communication in favor of better and more practical technologies. Today we have access to powerful computers as well as the internet. More practical, sure, but this also means that our previous malicious messenger has become much more powerful. He is now everywhere: the Wi-Fi in the Starbucks cafe you’re sitting in, the different servers making up the internet and forwarding your messages, the machines running our algorithms. Our enemies are now able to observe many more messages, as each request you make to a website might pass through the wrong wire, and become altered or copied in a matter of nanoseconds without anyone noticing.
Before us, we can see that old and recent history contains many instances of encryption algorithms falling apart, being broken by secret state organizations or by independent researchers, and failing to protect their messages or accomplish their claims. Many lessons were learned, and we slowly came to understand how to produce good cryptography.
![[Note]](https://drek4537l1klr.cloudfront.net/wong/v-12/Figures/note.png) Note
Note
A cryptographic algorithm can be considered broken in many ways. For an encryption algorithm, you can imagine several ways to attack the algorithm: the secret key can be leaked to the attackerm messages can be decrypted without the help of the key, some information about the message can be revealed just by looking at the encrypted message, and so on. Anything that would somehow weaken the assumptions we made about the algorithm could be considered a break.
A strong notion came out of the long process of trial and errors that cryptography went through: to obtain confidence in the security claims made by a cryptographic primitive, the primitive has to be analyzed in the open by experts. Short of that, you are relying on security through obscurity, which hasn’t worked well historically. This is why cryptographers (the people who build) will usually use the help of cryptanalysts (the people who break) in order to analyze the security of a construction. (Although cryptographers are often cryptanalysts themselves, and vice-versa.)

Let’s take the Advanced Encryption Standard (AES) encryption algorithm as an example. AES was the product of an international competition organized by the National Institute of Standards and Technology (NIST).
![[Note]](https://drek4537l1klr.cloudfront.net/wong/v-12/Figures/note.png) Note
Note
The NIST is a United States agency whose role is to define standards and develop guidelines for use in government-related functions as well as other public or private organizations. It has standardized many widely-used cryptographic primitives like AES.
The AES competition lasted several years, during which many volunteering cryptanalysts from around the world gathered to take a chance at breaking the various candidate constructions. After several years, once enough confidence was built by the process, a single competing encryption algorithm was nominated to become the Advanced Encryption Standard itself. Nowadays, most people trust that AES to be a solid encryption algorithm, and it is widely used to encrypt almost anything. For example, you use it every day when you browse the web.
The idea to build cryptographic standards in the open is related to a concept often referred to as Kerckhoffs' principle, which can be understood as: it would be foolish to rely on our enemies not to discover what algorithms we use, because they most likely will. Instead, let’s be open about them.
If the enemies of queen Alice and lord Bob knew exactly how they were encrypting messages, how was our encryption algorithm secure? The secret key! The secrecy of the key makes the protocol secure, not the secrecy of the algorithm itself. This is a common theme in this book: all the cryptographic algorithms that we will learn about, and that are used in the real-world, are most often free to be studied and used. Only the secret keys that are used as input to these algorithms, when being used, are kept secret. "Ars ipsi secreta magistro" (an art secret even to its inventor) said Jean Robert du Carlet in 1644.
In the next section, we will talk about a totally different kind of cryptographic primitive, so let’s use figure 1.4 to organize what we’ve learned so far.
Figure 1.4. The cryptographic algorithms we have learned so far. AES is an instantiation of a symmetric encryption algorithm, which is a cryptographic primitive that is part of the broader class of symmetric cryptographic algorithms. There are many more of those (represented as question marks) that we will learn about in this book.

highlight, annotate, and bookmark
You can automatically highlight by performing the text selection while keeping the alt/ key pressed.

In our discussion about symmetric encryption, we said that Alice and Bob first met to decide on a symmetric key. This is a plausible scenario, and a lot of protocols actually do work like this. Nonetheless, this quickly becomes less practical in protocols with many participants: do we need our web browser to meet with Google, Facebook, Amazon, and the other billions of websites before being able to securely connect to them? This problem, often referred to as key distribution has been a hard one to solve for quite a long time, at least until the discovery in the late 70s of another large and useful category of cryptographic algorithms called asymmetric cryptography or public-key cryptography. Asymmetric cryptography generally makes use of different keys for different functions (as opposed to a single key being used in symmetric cryptography), or provides different point of views to different participants. To illustrate what this mean, and how public-key cryptography helps to set up trust between people, I’ll introduce a number of asymmetric primitives in this section.
Note that this is only a glance of what you’ll learn in this book, as I’ll talk about each of these cryptographic primitives in more detail in subsequent chapters.
The first asymmetric cryptography primitive we’ll look at is the key exchange one.
The first public-key algorithm discovered and published was a key exchange algorithm named after its authors—Diffie-Hellman. The Diffie-Hellman key exchange algorithm’s main purpose is to establish a common secret between two parties. This common secret can then be used for different purposes, for example, as a key to a symmetric encryption primitive.
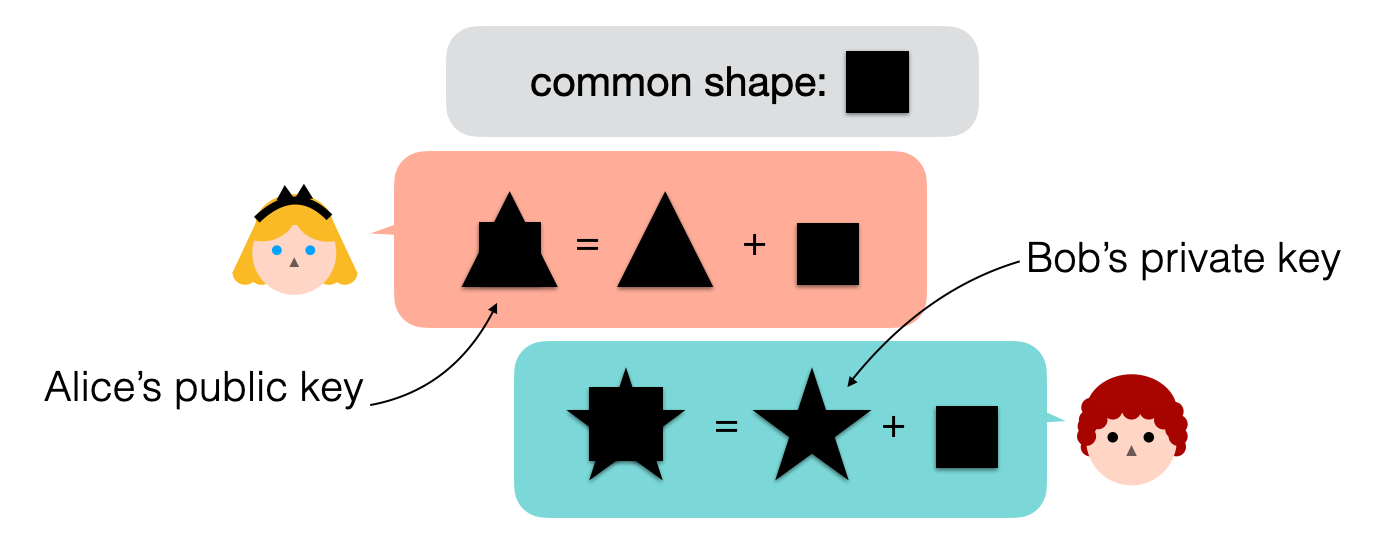
In chapter 6, I will explain in detail how Diffie-Hellman works, but for this introduction let’s use a simple analogy in order to understand what a key exchange provides. Like many algorithms in cryptography, a key exchange must start with the participants using a common set of parameters. In our analogy, we will simply have them agree to use a square ■.
The next step is for Alice and Bob to choose their own random shape. Both of them go to their respective secret place, and out of sight, Alice chooses a triangle ▲ and Bob chooses a star ★. The objects they have chosen need to remain secret at all costs! The objects represents their private keys (see figure 1.5).
Figure 1.5. The first step of a Diffie-Hellman key exchange is to have both participants generate a private key. In our analogy, Alice chooses a triangle as her private key, whereas Bob chooses a star as his private key.

Once they have done that, they both individually combine their secret shape with the common shape they initially agreed on using. This gives out a unique shape, which represents their public key. Alice and Bob can now exchange their public keys (hence the name "key exchange") as public keys are considered public information. I illustrate this in figure 1.6.
Figure 1.6. The second step of a Diffie-Hellman key exchange is to have both participants exchange their public keys. Participants derive their public keys by combining their private keys with the common shape.
We are now starting to see why this algorithm is called a public-key algorithm. It is because it requires a key pair: a private key and a public key.
The final step of the Diffie-Hellman key exchange algorithm is quite simple: Alice takes Bob’s public key, and combines it with her private key. Bob does the same with Alice’s public key, and combines it with his own private key. The result should now be the same on each side, in our example a shape combining a star, a square and a triangle (see figure 1.7).
Figure 1.7. The final step of a Diffie-Hellman key exchange is to have both participants produce the same shared secret. To do this, Alice combines her private key with Bob’s public key, and Bob combines his private key with Alice’s public key. The shared secret cannot be obtained from solely observing the public keys.

It is now up to the participants of the protocol to make use of this shared secret. We will see several examples of this in this book, but the most obvious use case is to make use of it in an algorithm that requires a shared secret. For example, Alice and Bob could now use the shared secret as a key to encrypt further messages with a symmetric encryption primitive.
To recap:
- Alice and Bob exchanged their public keys, which is masking their respective private keys.
- With the other participant’s public key, and their respective private key, they can compute a shared secret.
- An adversary who observes the public keys being exchanged doesn’t have enough information to compute the shared secret.
![[Note]](https://drek4537l1klr.cloudfront.net/wong/v-12/Figures/note.png) Note
Note
In our example, the last point is easily bypassable. Indeed, without the knowledge of any private keys we can combine the public keys together to produce the shared secret. Fortunately, this is only a limitation of our analogy, but it works well enough for us to understand what a key exchange does.
In practice, a Diffie-Hellman key exchange is quite insecure. Can you take a few seconds to figure out why?
As Alice will accept any public key she receives as being Bob’s public key, I could intercept the exchange and replace it with mine, which would allow me to impersonate Bob to Alice (and the same can be done to Bob). We say that a man in the middle (MITM) can successfully attack the protocol. How do we fix this? We will see in later chapters that we either need to augment this protocol with another cryptographic primitive, or we need to be aware in advance of what Bob’s public key is.
But then, aren’t we back to square one?
Previously Alice and Bob needed to know a shared secret, now Alice and Bob need to know their respective public keys. How do they get to know that? Is that a chicken-and-egg problem all over again? Well, kind of. As we will see, in practice public-key cryptography does not solve the problem of trust, but it simplifies its establishment (especially when the number of participants is large).
Let’s stop here and move on to the next section, as you will learn more about key exchanges in chapter 5. We still have a few more asymmetric cryptographic primitives to uncover (see figure 1.8), to finish our tour of real-world cryptography.
Figure 1.8. The cryptographic algorithms we have learned so far. Two large classes of cryptographic algorithms are symmetric cryptography (with symmetric encryption) and asymmetric cryptography (with key exchanges).

The invention of the Diffie-Hellman key exchange algorithm was quickly followed by the invention of the RSA algorithm named after Ron Rivest, Adi Shamir, and Leonard Adleman. RSA contains two different primitives: a public-key encryption algorithm (or asymmetric encryption) and a (digital) signature scheme. Both primitives are part of the larger class of cryptographic algorithms called asymmetric cryptography. In this section we will explain what these primitives do, and how they can be useful.
The first one, asymmetric encryption, has a similar purpose to the symmetric encryption algorithm we’ve talked about previously: it allows one to encrypt messages in order to obtain confidentiality. Yet, unlike symmetric encryption, which had the two participants encrypt and decrypt messages with the same symmetric key, asymmetric encryption is quite different:
- it works with two different keys: a public key and a private key.
- it provides an asymmetric point of view: anyone can encrypt with the public key, but only the owner of the private key can decrypt messages.
Let’s now use a simple analogy to explain how one can use asymmetric encryption. We start with our friend Alice again, who holds a private key (and its associated public key). Let’s picture her public key as an open chest that she releases to the public for anyone to use (see figure 1.9).
Figure 1.9. To use asymmetric encryption, Alice needs to first publish her public key (represented as an open box here). Now, anyone can use the public key to encrypt messages to her. And she should be able to decrypt them using the associated private key.

Now, you and I and everyone who wants can encrypt a message to her using her public key. In our analogy, imagine that you would insert your message into the open chest and then close it. Once the chest is closed, nobody but Alice should be able to open it. The box effectively protects the secrecy of the message from observers on the way.
The closed box (or encrypted content) can then be sent to Alice, and she can use her private key (only known to her, remember) to decrypt it (see figure 1.10).
Figure 1.10. Asymmetric Encryption: (1) anyone can use Alice’s public key to encrypt messages to her. (2) After receiving them, (3) she can decrypt the content using her associated private key. Nobody is able to observe the messages directed to Alice while they are being sent to her.

Let’s summarize in figure 1.11 the cryptographic primitives we have learned so far. We are only missing one more to finish our tour of real-world cryptography!
Figure 1.11. The cryptographic algorithms we have learned so far. Two large class of cryptographic algorithms are symmetric cryptography (with symmetric encryption) and asymmetric cryptography (with key exchanges and asymmetric encryption).

We’ve seen that RSA provides an asymmetric encryption algorithm, but as we’ve mentioned earlier, it also provides a digital signature algorithm.
![[Note]](https://drek4537l1klr.cloudfront.net/wong/v-12/Figures/note.png) Note
Note
The word "RSA" is often used to mean either RSA encryption or RSA signatures depending on context, which is always a great source of confusion. You’ve been warned.
The invention of this digital signature cryptographic primitive has been of immense help to set up trust between the Alices and Bobs of our world. It is similar to real signatures, you know, the one you are required to sign on a contract when you’re trying to rent an apartment, for example. "What if they forge my signature?" you may ask, and indeed real signatures don’t provide much security in the real-world. On the other hand, cryptographic signatures can be used in the same kind of way, but provide a cryptographic certificate with your name on it. Your cryptographic signature is unforgeable, and can easily be verified by others. Pretty useful compared to the archaic signatures you used to write on checks!
In figure 1.12, we can imagine a protocol where Alice wants to show David that she trusts Bob. This is a typical example of how to establish trust in a multi-participant setting, and how asymmetric cryptography can help. By signing a piece of paper containing "I, Alice, trust Bob," Alice can take a stance and notify David that Bob is to be trusted. If David already trusts Alice and her signature algorithm, then he can choose to trust Bob in return.
Figure 1.12. David already trusts Alice. Since Alice trusts Bob, can David safely trust Bob as well?

In more detail, Alice can use the RSA signature scheme and her private key to sign the message "I, Alice, trust Bob." This generates a signature that should look like random noise (see figure 1.13).
Figure 1.13. To sign a message, Alice uses her private key and generates a signature.

Anyone can then verify the signature, by combining:
- Alice’s public key.
- The message that was signed.
- The signature.
The result is either true (the signature is valid) or false (the signature is invalid) as can be seen in figure 1.14.
Figure 1.14. To verify a signature from Alice, one also needs the message signed and Alice’s public key. The result is either validating the signature, or invalidating it.

We have now learned about three different asymmetric primitives:
- Key exchange with Diffie-Hellman.
- Asymmetric encryption.
- Digital signatures with RSA.
These three cryptographic algorithms are the most known and commonly used primitives in asymmetric cryptography. It might not be totally obvious how these can help to solve real-world problems, but rest assured, they are used every day by many applications to secure things around them.
It is time to complete our picture with all the cryptographic algorithms we’ve learned about so far. See figure 1.15.
Figure 1.15. The symmetric and asymmetric algorithms we have learned so far.

discuss

In the previous section, we have surveyed two large classes of algorithms:
- Symmetric cryptography (or secret-key cryptography). A single secret is used. If several participants are aware of the secret, it is called a shared secret.
- Asymmetric cryptography (or public-key cryptography). Participants have an asymmetrical view of the secrets. For example, some will have knowledge of a public key, while some will have knowledge of both a public and private key.
Symmetric and asymmetric cryptography are not the only two categories of primitives in cryptography, and it’s quite hard to classify the different subfields. But yet, as you will realize, a large part of our book is about (and makes use of) symmetric and asymmetric primitives. This is because a large part of what is useful in cryptography, nowadays, is contained in these subfields.
Another way of dividing cryptography can be:
- Math-based constructions. These are constructions that rely on mathematical problems like factoring numbers. (The RSA algorithm for digital signatures and asymmetric encryption is an example of such a construction.)
- Heuristic-based constructions. These are the constructions that rely on observations and statistical analysis by cryptanalysts. (AES for symmetric encryption is an example of such a construction.)
There is also a speed component to this categorization, as mathematic-based constructions are often much slower than heuristic-based constructions. To give you an idea, symmetric constructions are most often based on heuristics (what seems to be working), while most asymmetric constructions are based on mathematical problems (what is thought to be hard).
It is hard for us to rigorously categorize all of what cryptography has to offer. Indeed, every book or course on the subject gives different definitions and classifications. In the end, these distinctions are not too useful for us, as we will see most of the cryptographic primitives as unique tools that make unique security claims. Many of these tools can in turn be used as building blocks to create protocols. It is thus essential to understand how each of these tools work, and what kind of security claims they provide, in order to understand how they provide security in the protocols around us. For this reason, the first part of this book will go through the most useful cryptographic primitives and their security properties.
A lot of the concepts in the book can be quite complicated the first time around. But like everything, the more we read about them, and the more we see them in context, the more natural they become. The more we abstract them. The role of this book is to help you to create these abstractions, to allow you to create a mental model of what these constructions do, and how they can be combined together to produce secure protocols. I will often talk about the interface of constructions, and give real-world examples of usage and composition.
The definition of cryptography used to be simple: Alice and Bob want to exchange secret messages. It isn’t anymore. What cryptography is nowadays, is quite complex to describe, and has grown organically around discoveries, breakthroughs and practical needs. At the end of the day, cryptography is what helps to augment a protocol, in order to make it work in adversarial settings.
To understand exactly how cryptography can help, the set of goals that these protocols want to achieve is what matters to us. That’s the useful part. Most of the cryptographic primitives and protocols we’ll learn about in this book provide one or two of the following properties:
- Confidentiality. It’s about masking and protecting some information from the wrong eyes. For example, encryption masks the messages in transit.
- Authentication. It’s about identifying who we are talking to. For example, this can be helpful in making sure that messages we receive indeed come from Alice.
Of course, it’s still a heavy simplification of what cryptography can provide. In most cases, the details are in the security claims of the primitives. Depending on how a cryptographic primitive is used in a protocol, it will achieve different security properties.
Throughout this book, we will learn new cryptographic primitives and how they can be combined to expose security properties like confidentiality and authentication. For now, appreciate the fact that cryptography is about providing insurances to a protocol in adversarial settings. While the "adversaries" are not clearly defined, we can imagine that they are the ones who attempt to break your protocol: a participant, an observer, a man in the middle. They reflect what a real life adversary could be. Because eventually, cryptography is a practical field made to defend against bad actors in flesh and bones and bits.
settings

Take our tour and find out more about liveBook's features:
- Search - full text search of all our books
- Discussions - ask questions and interact with other readers in the discussion forum.
- Highlight, annotate, or bookmark.
In 1993, Schneier releases Applied Cryptography, a book targeting developers and engineers who want to build applications that involve cryptography. Circa 2012, Kenny Paterson and Nigel Smart start an annual conference called Real World Crypto, targeting the same crowd and taking place every year. But what do applied cryptography and real-world cryptography refer to? Is there more than one type of cryptography?
To answer the question, we have to start by defining theoretical cryptography, the cryptography that cryptographers and cryptanalysts work on. These crypto people are mostly from the academia, working in universities, but sometimes from the industry or in specific departments of the government. They research everything and anything in cryptography. Results are shared internationally through publications and presentations in journals and conferences. Yet, not everything they do is obviously useful or practical. Often, no "proof of concept" or code is released, and it wouldn’t make sense anyway as no computer is powerful enough to run their research. Having said that, theoretical cryptography does sometimes become so useful and practical that it makes its way to the other side.
The other side is the the world of applied cryptography, or real-world cryptography. It is the foundation of the security you find in all applications around you. Although it often seems like it’s not there, almost transparent, it is here when you log into your bank account on the internet, it is with you when you message your friends, it helps protect you when you lose your phone. It is ubiquitous, because unfortunately attackers are everywhere and actively do try to observe and harm our systems. Practitioners are usually from the industry, but will sometimes vet algorithms and design protocols with the help of the academia community. Results are often shared through conferences, blog posts, and open-source software.
Real-world cryptography usually cares deeply about real-world considerations: what is the exact level of security provided by an algorithm? How long does it take to run the algorithm? What is the size of the inputs and outputs required by the primitive? Real-world cryptography is, as you might have guessed, the subject of this book. While theoretical cryptography is the subject of other books, we will still take a peek at what is brewing there in the last chapters of this book. Be prepared to be amazed, as you might catch a glance of the real-world cryptography of tomorrow.
Now you might wonder: how do developers and engineers choose what cryptography to use for their real-world applications?
highlight, annotate, and bookmark
You can automatically highlight by performing the text selection while keeping the alt/ key pressed.

| Sitting on top are cryptanalysts who propose and solve hard mathematical problems […] and at the bottom are software engineers who want to encrypt some data. |
||
| -- Thai Duong So you want to roll your own crypto? (2020) | ||
In all those years I’ve spent studying and working with cryptography, I’ve never noticed a single pattern in which a cryptographic primitive ends up being chosen to be used in real-world applications. Things are pretty chaotic, before a theoretical primitive gets to be adopted there’s a long list of people who get to handle the primitive and shape it into something consumable and sometimes safer for the public at large. So how can I even explain that to you… Have you heard of Choose Your Own Adventure? It’s an old book series that got you to pick how you wanted to step through the story. The principle was simple: you read the first section of the book; at the end of the section, the book lets you decide on the path forward by giving you different options; each option was associated to a different section number that you could skip directly to if you so chose. So I’ve done the same! Start by reading the next paragraph, and follow the direction it gives you.
Where it all begins. Who are you? Are you Alice, a cryptographer? Are you David, working in the private industry and in need of a solution to your problems? Or are you Eve, working in a government branch and preoccupied by cryptography?
- You’re Alice, go to step 1.
- You’re David, go to step 2.
- You’re Eve, go to step 3.
Step 1 - Researchers gotta research. You’re a researcher working in a university, or in the research team of a private company or a non-profit, or in a government research organization like the NIST or the NSA. As such, your funding can come from different places and might incentivize you to research different things.
- You invent a new primitive, go to step 4.
- You invent a new construction, go to step 5.
- You start an open competition, go to step 6.
Step 2 - The industry has a need. As part of your job, something comes up, and you are in need of a new standard. For example, the Wi-Fi Alliance is a non-profit funded by interested companies to produce the set of standards around the Wi-Fi protocol. Another example are banks, that got together to produce the Payment Card Industry Data Security Standard (PCI-DSS) which enforces algorithms and protocols to use if you deal with credit card numbers.
- You decide to fund some much-needed research, go to step 1.
- You decide to standardize a new primitive or protocol, go to step 5.
- You start an open competition, go to step 6.
Step 3 - A government has a need. You’re working for your country’s government, and you need to push out some new crypto. For example, the NIST is tasked with publishing the Federal Information Processing Standards (FIPS) which mandates what cryptographic algorithms can be used by companies that deal with the US government. While many of these standards were success stories, and people tend to have a lot of trust in standards being pushed by government agencies, there is unfortunately a lot to say about failures. In 2013, it was discovered following revelations from Edward Snowden, that the NSA had purposefuly and successfully pushed for the inclusion of backdoors algorithms in standards (see "Dual EC: A Standardized Back Door" by Bernstein et al.). which included a secret switch that allowed the NSA and only the NSA to predict your secrets). These backdoors can be thought as magic passwords that allow the government (and only them, suposedly) to subvert your encryption. Following this, the cryptographic community has lost a lot of confidence in standards and suggestions coming from government bodies. Recently, in 2019, it was found that the russian standard GOST had been victim of the same treatment.
| Cryptographers have long suspected that the agency planted vulnerabilities in a standard adopted in 2006 by the National Institute of Standards and Technology and later by the International Organization for Standardization, which has 163 countries as members. Classified N.S.A. memos appear to confirm that the fatal weakness, discovered by two Microsoft cryptographers in 2007, was engineered by the agency. The N.S.A. wrote the standard and aggressively pushed it on the international group, privately calling the effort "a challenge in finesse. |
||
| -- New York Times N.S.A. Able to Foil Basic Safeguards of Privacy on Web (2013) | ||
- You fund some research, go to step 1.
- You organize an open competition, go to step 6.
- You push for the standardization of a primitive or protocol that you’re using, go to step 7.
Step 4 - A new concept is proposed. As a researcher, you manage to do the impossible, you invent a new concept. Sure someone already thought about encryption, but there are still new primitives being proposed every year in cryptography. Some of them will prove to be impossible to realize, and some of them end up being solvable. Maybe you have an actual construction as part of your proposal, or maybe you’ll have to wait to see if someone can come up with something that works.
- Your primitive gets implemented, go to step 5.
- Your primitive ends up being impossible to implement, go back to the beginning.
Step 5 - A new construction or protocol is proposed. A cryptographer, or team of cryptographers, proposes a new algorithm that instantiates a concept. For example, AES is an instantiation of an encryption scheme. AES was initially proposed by Vincent Rijmen and Joan Daemen (who named their construction as a constraction of their names, Rijndael). What’s next?
- Someone builds on your construction, go to step 5.
- Your partake in an open competition and win! Go to step 6.
- There’s a lot of hype for your work, you’re getting a standard! Go to step 7.
- You decide to patent your construction, go to step 8.
- You, or someone else, decides that it’ll be fun to implement your construction. Go to step 9.
Step 6 - An algorithm wins a competition. The process cryptographers love the most is an open competition! For example, the Advanced Encryption Standard (AES) was a competition that invited researchers from all over the world to compete. After dozens of submissions and rounds of analysis and help from cryptanalysts (which can take years), the list was reduced to a few candidates (in the case of AES, a single one), which then moved to become standardized.
- You got lucky, after many years of competition your construction won! Go to step 7
- Unfortunately, you lost. Go back to the start.
Step 7 - An algorithm or protocol is standardized. A standard is usually published by a government or by a standardization body. The aim is to make sure that everyone is on the same page, so as to maximalize interoperability. For example, the NIST (a US government organization) regularly publishes cryptographic standards. A well-known standardization body in cryptography is the Internet Engineering Task Force (IETF), which is behind many standards on the internet (like TCP, UDP, TLS, and so on), and that you will hear about a lot in this book. Standards in the IETF are called Request For Comment (RFC), and can be written by pretty much anyone who wants to write a standard.
| To reinforce that we do not vote, we have also adopted the tradition of "humming": When, for example, we have face-to-face meetings and the chair of the working group wants to get a "sense of the room", instead of a show of hands, sometimes the chair will ask for each side to hum on a particular question, either "for" or "against". |
||
| -- RFC 7282 On Consensus and Humming in the IETF (2014) | ||
Sometimes, a company publishes a standard directly. For example, RSA Security LLC (funded by the creators of the RSA algorithm), released a series of 15 documents called the Public Key Cryptography Standards (PKCS), in order to legitimize algorithms and techniques the company was using at the time. Nowadays, this is pretty rare and a lot of companies will go through the IETF to standardize their protocols or algorithms as an RFC, instead of a custom document.
- Your algorithm or protocol gets implemented, go to step 9.
- Nobody cares about your standard, go back to the start.
Step 8 - A patent expires. A patent in cryptography usually means that nobody will use the algorithm. Once the patent expired, it is not uncommon to see a renewed interest in the primitive. The most popular example is probably Schnorr signatures, which were the first contender to become the most popular signature scheme until Schnorr himself patented the algorithm in 1989. This led to the NIST standardizing a poorer algorithm (DSA), which became (at the time) the go-to signature scheme. The patent over Schnorr signatures has expired in 2008, and the algorithm has since started regaining popularity.
- It’s been too long, your algorithm will be forever forgotten. Go back to the beginning.
- Your constructions inspires many more constructions to get invented on top of it, go to step 5.
- Now people want to use your construction, but not before it’s standardized for real. Go to step 7.
- Some developers are implementing your algorithm! Go to step 9.
Step 9 - A construction or protocol gets implemented. Implementers have the hard task to not only decipher a paper or a standard (although standards are supposed to target implementers, "supposed”), but they also must make their implementations easy and safe to use. This is not always an easy task, as many devastating bugs can arise in the way cryptography is used.
- Someone decides it is time for these implementations to be backed by a standard. It’s embarassing without one. Go to step 7.
- Hype is raining on your cryptographic library! Go to step 10.
Step 10 - A developer uses a protocol or primitive in an application. A developer has a need, and your cryptographic library seems to solve it. Easy as!
- The primitive solves the need, but they don’t have a standard… Not great. Go to step 7.
- I wish this was written in my programming language. Go to step 9.
- I misused the library, or the construction is broken. Game over.
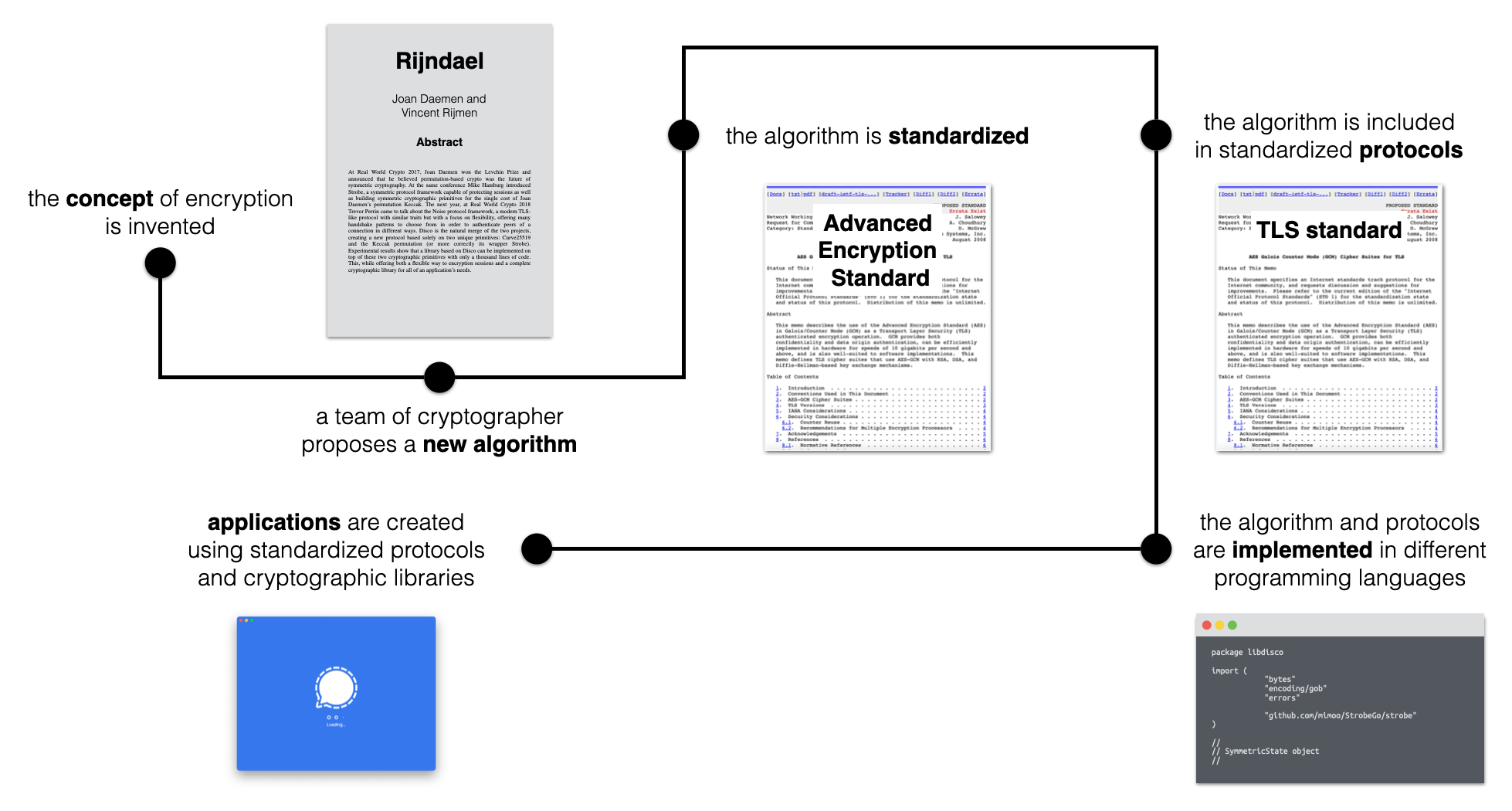
You got it: there’s many means for a primitive to go real-world. The best way involves many years of analysis, a good standard, and good libraries; A bad way involves a bad algorithm with a bad implementation. In figure 1.16 I illustrate the preferred path.
Figure 1.16. The ideal lifecycle for a cryptographic algorithm: it starts when cryptographers instantiate a concept in a whitepaper. For example, AES is an instantiation of the concept of symmetric encryption (there are many more symmetric encryption algorithms out there). A construction can then be standardized: everybody agree to implement it a certain way to maximize interoperability. Then support is created by implementing the standard in different languages.
discuss

| Anyone, from the most clueless amateur to the best cryptographer, can create an algorithm that he himself can’t break. |
||
| -- Bruce Schneier Memo to the Amateur Cipher Designer (1998) | ||
I must warn you, the art of cryptography is difficult to master and it would be unwise to build complex cryptographic protocols once you’re done with this book.
This journey should enlighten you, show you what is possible, and show you how things work, but it will not make you a master of cryptography. This book is not the holy grail. Indeed, the last pages of this book will have you go through the most important lesson: do not go alone on a real adventure. Dragons can kill, and you need some support to accompany you in order to defeat them. In other words, cryptography is complicated, and this book alone does not permit you to abuse what you learn. To build complex systems, experts who have studied their trade for years are required. Instead, what you will learn is to recognize when cryptography should be used or if something seems fishy, what cryptographic primitives and protocols are available to solve the issues you’re facing, and how all these cryptographic algorithms work under the surface.
Now that you’ve been warned, go to the next chapter.
settings

- A protocol is a step by step recipe where multiple participants attempt to achieve something, like exchanging confidential messages.
- Cryptography is about augmenting protocols to secure them in adversarial settings. It often requires secrets.
- A cryptographic primitive is a type of cryptographic algorithm. For example, symmetric encryption is a cryptographic primitive, while AES is a specific symmetric encryption algorithm.
- One way to classify the different cryptographic primitives is to split them into two types: symmetric and asymmetric cryptography: symmetric cryptography uses a single key (as you’ve seen with symmetric encryption), while asymmetric cryptography makes use of different keys (as you’ve seen with key exchanges, asymmetric encryption, and digital signatures).
- Cryptographic properties are hard to classify, but they often aim to provide one of these two properties: authentication or confidentiality. Authentication is about verifying the authenticity of something or someone, while confidentiality is about the privacy of data or identities.
- real-world cryptography matters because it is ubiquitous in technological applications, while theoretical cryptography is often less useful in practice.
- Most of the cryptographic primitives contained in this book were agreed on after long standardization processes.
- Cryptography is complicated and there are many dangers in implementing or using cryptographic primitives.